Tags: #discountcashflow, #Netpresentvalue, #Timevalueofmoney, #financialmodels, #hospitalitycode, #DCF, #NPV, #TVM

In this series of articles, I will cover key financial models and this is the second article on the subject. I will cover three related concepts; Discounted Cash Flow, Net Present Value and Time Value of Money.
Time value of money concepts are the cornerstone of modern finance
Definitions of Time Value of Money and Discounted Cash Flow
The time value of money (TVM) is the idea that money available at the present time is worth more than the same amount in the future due to its potential earning capacity. This core principle of finance holds that provided money can earn interest, any amount of money is worth more the sooner it is received.
Martin de Azpilcueta of School of Salamanca (1491 – 1586), also known as Doctor Navarrus is the inventor of Time Value of Money Concept.
In modern finance, time value of money concepts plays a central role in decision support and planning. When investment projections or business case results extend more than a year into the future, it is important to see the time value of money impact on long-term projections and therefore, we need to see cash flows in both with discounting and without discounting forms.
Discounted cash flow DCF is an application of the time value of money concept—the idea that money to be received or paid at some time in the future has less value, today, than an equal amount actually received or paid today.
The time value of money (TVM) is the idea that money available at the present time is worth more than the same amount in the future due to its potential earning capacity
One of the reasons we are reviewing DCF and TVM together is the interlink between the concepts. When we analysing Discounted cash flow analysis DCF, the two-time value of money terms is the key;
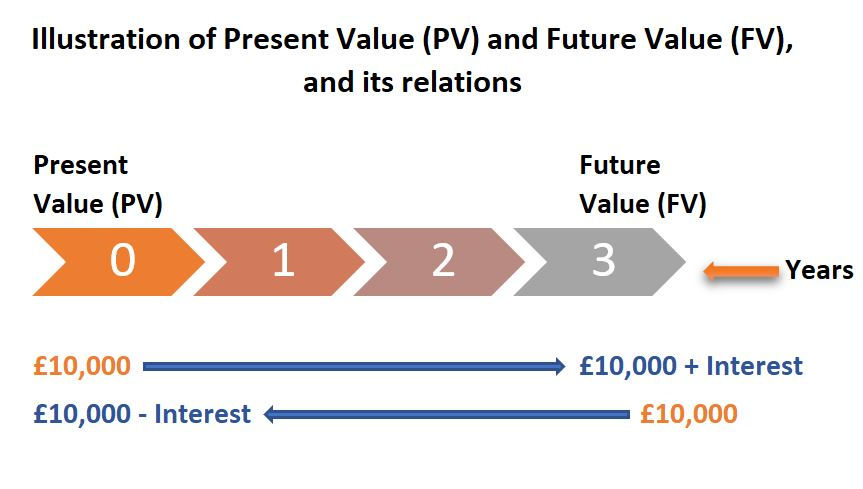
Present value (PV) is what the future cash flow is worth today.
Future value (FV) is the value that actually flows in or out at the future time.
The DCF calculation finds the value appropriate today—the present value—for the future cash flow. A £100 cash inflow that will arrive two years from now could, for example, have a present value today of about £95, while its future value is by definition £100.
Discount Cash Flow (DCF) can be an important factor when evaluating or comparing investments, action proposals, or purchases.
Is Time Value of Money Real Value?
Time value of money concepts are easier to understand when explained together and I will try to do it as easier as possible.
Let’s accept it, when we all here “Time Value of Money” the first time, it sounds like fiction and comparing to the cold, serious and calculated face of Finance it feels unreal.
Having the use of money for a specific period of time has a value that is tangible, measurable, and real
Time Value of Money as a concept has two separate applications; discounted cash flow and interest paid for a loan.
DCF – Discounted Cash Flow; the discounting lowers the present value (PV) of future funds. What future money is worth today is called its present value (PV) and what it will be worth in the future when it finally arrives is called not surprisingly its future value (FV). Present value, in other words, is discounted below future value.
There are three factors impact future value (FV) of our today’s money;
Inflation; inflation over time reduces the buying power of money.
Risk; money you have today is real but future is less certain which creates a risk factor
Opportunity; if you invest your money today, you could get a better return somewhere in the future. The money you will not have until a future time cannot be used now.
What is Cash Flow Stream and How does it work with different Discount Rates over time?
A series of cash inflows or outflows coming at different future times, the series is called a cash flow stream
Each future cash flow has its own value today (its own present value). The sum of these present values is the net present value of the cash flow stream.
I will try to explain this with an example; consider an investment today of £100, that brings net gains of £100 each year for 5 years. To be able to compare and contrast I added two different discount rates (interest rate); 8% and 15%. The future values and present values of these cash flow events might look like this:

The first blue bars represent cash flow figures for each year and appear when they actually appear in the future (Future Values – FV).
Orange represents the discount rate of 8% and Grey represents the discount rate of 15% values of the same cash flows in present value (PV) terms.
If you look at the legend area, the net cash flow expected after five years is £400. However, the net present value (NPV) today is discounted to something to something less. So, NPV at 8% interest rate is £299 and 15% is £235.
I would like to draw your attention that when we apply longer term and higher interest rates, the present value of our cash decreases.
When we calculating Net Present Value, the amount of time between now and each future payment period and discount (interest) rate impacts the size of the discounting effect. We will review the role of interest rate and time periods when we determining net present values in mathematic terms.
Key Future Value (FV), Present Value (PV) and Net Present Value (NPV) Calculations
The term used to identify this is Calculations for interest growth and compounding. Let’s start with easy examples and we can dig deeper as we go along.
Before we start, I would like to give explanations of some of the abbreviations we will use;
FV = Future Value
PV = Present Value
i = Discount (Interest) Rate
n = Number of Periods
NPV = Net Present Value
q = Number of periods per year
CF = Cash Flow
DCF = Discounted Cash Flow
Example-1: What is the future value (FV) in one year, of £1,000 invested today (the PV), at an annual interest rate of 15%?

Answer: FV1 = £1,000 x (1 + 0.15) 1 = £1,150
When the Future Value is more than one period into the future, interest compounding takes place. Interest compounding means, interest earned in earlier periods begins to earn interest on itself, in addition to interest on the original Present Value.
Example-2: What is the future value in five years of £1,000 invested today at an annual interest rate of 15%?
Answer: FV5 = £1,000 x (1 + 0.15)5 = £2,011
So far, we look at the future value of our money at hand; let’s start from the future and looking backwards in time, to today. Instead of Future Value (FV) of current money, let’s find Present Value (PV) of a future money.
Example-3: What is the value today of a £1,000 payment arriving in one year, using an interest rate of 15%?

Answer: PV1 = £1,000 / (1 + 0.15) 1 = £870
Example-4: What is the present value of £1,000 we will receive in 5 years, using a 15% interest rate?
Answer: PV5 = £1,000 / (1 + 0.15) 5 = £497
All the examples we used so far assuming period-end (year-end) discounts, all our calculators and excel functions also designed the same way.
NPV = (FV0 / (1+i)0) + (FV1 / (1+i)1) + …. + (FV n / (1+i)n)
There is also another method, called Mid-period discounting. As we mentioned earlier, the discount rate recognizes the values of opportunity, risk, and inflation—values that change continuously as time passes. Mid-period discounting applies the discounting effect precisely when cash actually flows and therefore more precise comparing to period-end discounting.
There are two formulations to apply;
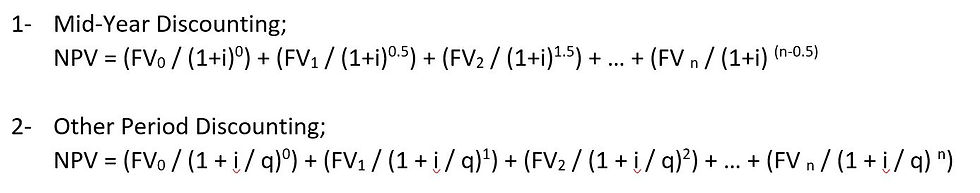
How to Compare two different Investment Opportunities with DCF and NPV
Exercise: There are two competing projects for your money. Both require an initial cash outlay of £1,000, and each returns a total of £2,250 over the five years period and making a net gain of £1,250. Considering both investment project requires similar investment and returns are the same, which project you should invest? (Note: 8% Interest Rate applied to both project)
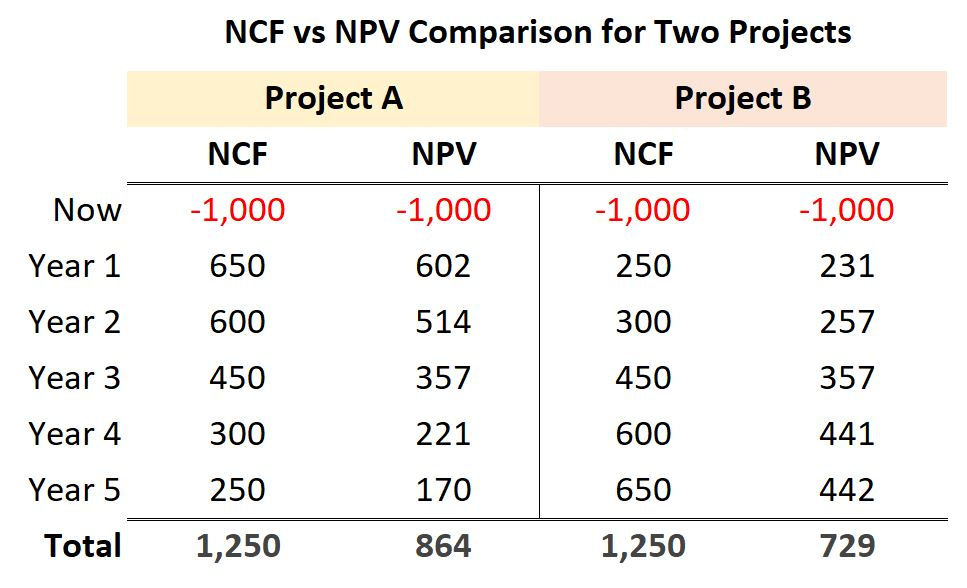
While total gains equal in both project on Net Cash Flow, the timing of the returns are different. As a result, the present value of each year’s return is different.
Comparing the two investments, the larger early returns in Project A lead to a better net present value (NPV) than the later large return in Project B. Therefore, other things being equal, Project A’s higher NPV return presents a better investment opportunity.
In summary, Net Present Value (NPV) and Discounted Cash Flow (DCF) are useful for investment analysis;
Different uses for money are being compared
The business case covers long periods of time (two or more years).
Inflows and outflows change differently over time (e.g., the largest inflows come at a different time from the largest outflows).
Two or more alternative cases are being compared and they differ with respect to cash flow timing within the analysis period.
Quick Guide to Calculator Financial Keys and Excel Function
While we reviewing the calculations, all financial calculators have five financial keys, and Excel's basic time value functions are exactly analogous. The table below shows the equivalency between the calculator keys and Excel functions for your usage:

Just as you have to supply at least three of the variables to solve a TVM problem in a financial calculator, you also have to supply at least three of the arguments to each Excel function. Note that in the table, the bold function arguments are required while those in italics are optional.
You don't need to memorize the order of the function arguments. You can use the Insert Function dialogue box, which will prompt you for the arguments by name. Or, if you prefer to type the function directly, Excel will display a Smart Tag that shows the order of the arguments as you type.
I have added downloadable free Excel file on our "Resource Hub" which Key Financial Models and its easy calculation samples for your use. Please visit hospitalitycode.com/resource-hub to download.
Please follow me on www.hospitalitycode.com for my articles on other financial models as well as other areas of Hospitality business, trends and interpretation methods.


コメント